High Speed Flight

• At low flight speeds the study of aerodynamics is greatly simplified by the fact that air may experience relatively small changes in pressure with only negligible changes in density. The airflow is termed incompressible since the air may undergo changes in pressure without apparent changes in density. Such a condition of airflow is analogous to the flow of water, hydraulic fluid, or any other incompressible fluid. However, at high flight speeds the pressure changes that take place are quite large and significant changes in air density occur. The study of airflow at high speeds must account for these changes in air density and must consider that the air is compressible and that there will be "compressibility effects."
• A factor of great importance in the study of high speed airflow is the speed of sound. The speed of sound is the rate at which small pressure disturbances will be propagated through the air and this propagation speed is solely a function of air temperature.
• As an object moves through the air mass, velocity and pressure changes occur which create pressure disturbances in the airflow surrounding the object. Of course, these pressure disturbances are propagated through the air at the speed of sound. If the object is traveling at low speed the pressure disturbances are propagated ahead of the object and the airflow immediately ahead of the object is influenced by the pressure field on the object. Evidence of this "pressure warning" is seen in the typical subsonic flow pattern of figure 3 where there is upwash and flow direction change well ahead of the leading edge. If the object is traveling at some speed above the speed of sound the airflow ahead will not be influenced by the pressure field on the object since pressure disturbances cannot be propagated ahead of the object. Thus as the flight speed nears the speed of sound a compression wave will form at the leading edge and all changes in velocity and pressure will take place quite sharply and suddenly.
• It is important to note that compressibility effects are not limited to flight speeds at and above the speed of sound. Since any aircraft will have some aerodynamic shape and will be developing lift there will be local flow velocities on the surfaces which are greater than flight speed. Thus, an aircraft can experience compressibility effects at flight speeds well below the speed of sound. Since there is a possibility of having both subsonic and supersonic flows existing on the aircraft it is convenient to define certain regimes of flight. These regimes are defined approximately as follows:
• Subsonic — Mach numbers below 0.75
• Transonic — Mach numbers from 0.75 to 1.20
• Supersonic — Mach number from 1.20 to 5.00
• Hypersonic — Mach numbers above 5.00
• The behavior of supersonic compressible flow is that a convergence causes compression while a divergence causes expansion. Thus, as the supersonic compressible flow approaches a constriction and the streamlines converge, velocity decreases and static pressure increases.

Source: Hurt, pp. 201-205

The equivalent airspeed (EAS) is the result of correcting the (CAS) for compressibility effects. At high flight speeds the stagnation pressure recovered in the pitot tube is not representative of the airstream dynamic pressure due to a magnification by compressibility Compressibility of the airflow produces a stagnation pressure in the pitot which is greater than if the flow were incompressible. As a result, the airspeed indication is given an erroneous magnification. The standard airspeed indicator is calibrated to read correct when at standard sea level conditions and thus has a compressibility correction appropriate for these conditions.

Source: Hurt, pp. 11-12

• One further correction must be made to obtain true airspeed. The airspeed indicator measures dynamic pressure which is:
$$q=\frac{\sigma V^2}{295}$$
• As altitude increases, the density ratio σ decreases. If the airspeed indicator is calibrated for sea level density ratio, it will be in error at all altitudes where sigma is not 1.0, and it will read values that are less than the true airspeed. In some airspeed indicators there is a barometric compensating device, and true airspeed is read directly from the instrument.
• True Airspeed (TAS), coupled with ambient density ratio, produces the same dynamic pressure as EAS, coupled with standard sea level density ratio. That is:
$$\left({\mathrm{TAS}}^2\right)\sigma =\left({\mathrm{TAS}}^2\right){\sigma }_0$$
• But σ₀ = 1.0, so
$$\mathrm{TAS}=\mathrm{EAS}\sqrt{1/\sigma }$$

Source: Dole, pp. 24-25

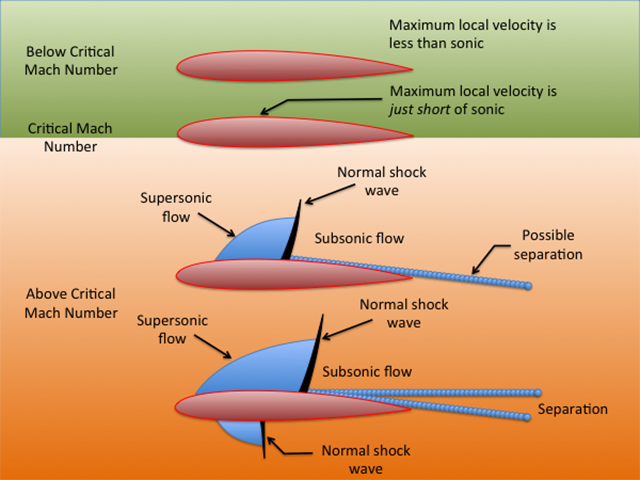
• If the flight Mach number increases, so does the local velocity on top of the wing. At some flight Mach number the local maximum velocity reaches sonic speed, M = 1.0. This flight Mach number is called the critical Mach number.
• Once M_CRIT is exceeded, the aircraft is flying in the transonic speed range. Supersonic airflow exists in the area of maximum thickness on top of the wing, subsonic flow exists elsewhere.
• As the air flows through the normal shock wave it undergoes a rapid compression. The compression decreases the kinetic energy of the airstream and converts it into a pressure and temperature increase behind the shock wave. The heat rise behind the shock wave is either radiated to the atmosphere or absorbed by the wing surface, but in either case it is lost, and this lost energy must be continuously supplied by the engines. This energy loss represents a type of drag known as wave drag.

Source: Dole, p. 217

• In order to obtain a high critical Mach number from an airfoil at some low lift coefficient the section must have:
1. Low thickness ratio. The point of maximum thickness should be aft to smooth the pressure distribution.
2. Low camber. The mean camber line should be shaped to help minimize the local velocity peaks.
• If supersonic flight is a possibility the thickness ratio and leading edge radius must be small to decrease wave drag.
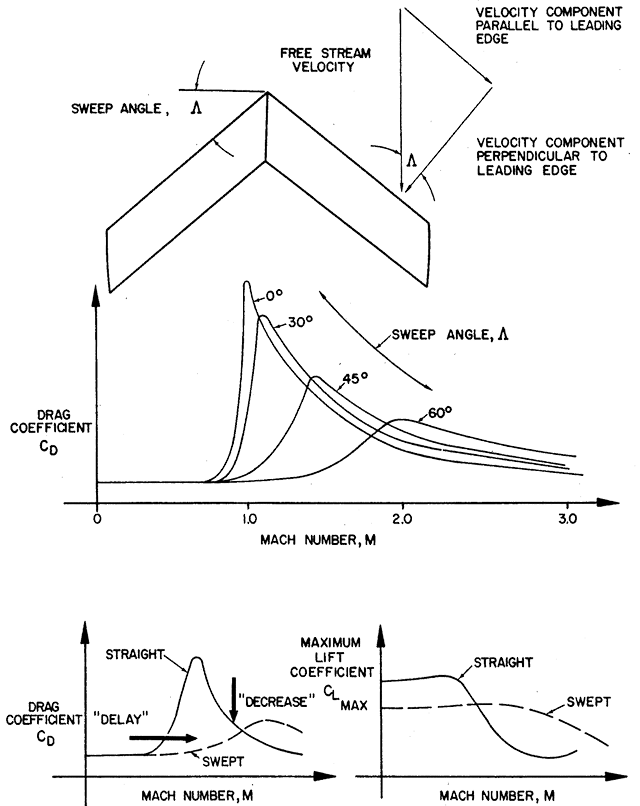
• Sweepback produces an unusual effect on the high speed characteristics of a surface and has basis in a very fundamental concept of aerodynamics. The swept wing shown has the streamwise velocity broken down to a component of velocity perpendicular to the leading edge and a component parallel to the leading edge. The component of speed perpendicular to the leading edge is less than the free stream speed (by the cosine of the sweep angle) and it is this velocity component which determines the magnitude of the pressure distribution.
• The component of speed parallel to the leading edge could be visualized as moving across constant sections and, in doing so, does not contribute to the pressure distribution on the swept wing. Hence, sweep of a surface produces a beneficial effect in high speed flight since higher flight speeds may be obtained before components of speed perpendicular to the leading edge produce critical conditions on the wing. This is one of the most important advantage of sweep since there is an increase in critical Mach number, force divergence Mach number, and the Mach number at which the drag rise will peak. In other words, sweep with delay the onset of compressibility effects.

Source: Hurt, pg. 223

• A wing produces lift by accelerating the air which passes over the top surface to a higher speed than that which passes under the bottom surface. The greater the difference between these two speeds the higher to difference in pressure, hence the larger the lift vector.
• Because the local speed of the upper surface air is higher than the free stream speed — a good bit higher where the camber is marked — it is clear that this airstream will become sonic before the effect first become apparent free stream reaches the sonic value. At this speed local shock waves are formed on the wing and compressibility effects become apparent; the drag increases, buffeting is felt and changes in lift and the position of the centre of pressure occur which, at a fixed tail angle, are reflected as changes in pitch. The Mach number at which these compressibility effects first become apparent is the critical Mach number; all other parameters aside, this can be quite low for a straight wing, around 0.7 Mach number.
• By sweeping a wing significantly, it will be remembered, the velocity vector normal to the leading edge is made less than the chord wise resultant. In [the figure] AC is shorter than AB. As the wing is responsive only to the velocity vector normal to the leading edge, for a given Mach number the effective chord wise velocity is reduced (in effect the wing is persuaded to believe that it is flying slower than it really is). This means that the airspeed can be increased before the effective chord wise component become sonic and thus the critical Mach number is raised.

Source: Davies, pg. 96-98

With the advent of the big jet three things occurred to make life even more difficult. The size of the aeroplane increased enormously and, with increase altitude capability, the speed increase difficulty was compounded by Mach number effects which caused pitch changes on the aeroplane, upset the pressure distribution over the control surfaces and brought about unwanted changes in hinge moments. It was about this time that the design of the pure aerodynamic control was seen to be, perhaps not impossible, but certainly very difficult, costly and time consuming. Something was needed to remove the problems — and the answer lay in operating the control surface by pure power.

Source: Davies, pg. 24